this post was submitted on 08 Oct 2024
1118 points (98.3% liked)
People Twitter
5268 readers
725 users here now
People tweeting stuff. We allow tweets from anyone.
RULES:
- Mark NSFW content.
- No doxxing people.
- Must be a tweet or similar
- No bullying or international politcs
- Be excellent to each other.
founded 1 year ago
MODERATORS
you are viewing a single comment's thread
view the rest of the comments
view the rest of the comments
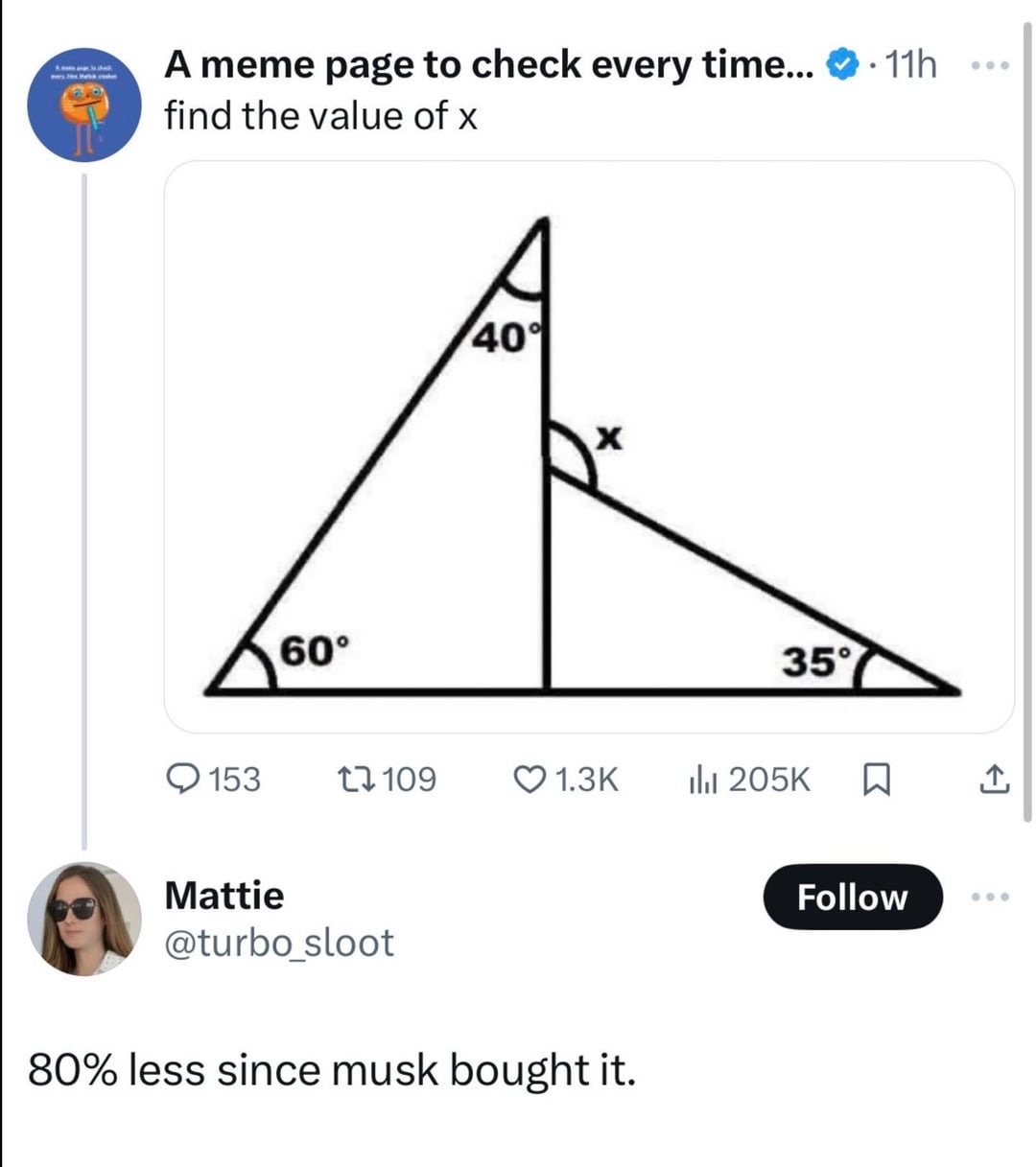
Except for the part where it's a single straight line segment, as depicted in the image. Showing the complimentary angles as an unlabeled approximately right angle is within convention. Showing a pair of line segments that do not form a straight line as a straight line is not.
Exactly.
Add to this that
xis literally impossible to calculate if conventions are not assumed, and absolutely possible to calculate if conventions are followed. Assuming the conventions won't hold is an irrational position.What you say makes no sense.
The problem is LITERALLY unsolvable if we can't assume that all the lines are straight.
The schematic was OF COURSE purposefully drawn in a way to make the viewer assume that the third angle of the left triangle is 90°, making the angle to it's right also be 90°, but the point of the exercise is to get the student to use ALL the given information instead of presuming right angles.
And NO, assuming all the lines are straight is NOT unreasonable, it is the only way that the problem could ever possibly have a solution.
I'd say that the shape on the left has what appears to be a little kink right near X, so one might infer that the shape on the left might be a quadrilateral. There are blatantly obvious vertices that are not labeled as such, so we can't assume that the not-quite-straight line is supposed to be straight since other angles are also not explicitly indicated as vertices...
Reasonable assumptions are a fundamental requirement for communication. It's not that you are wrong in what you are saying. There is a chance that the poser of the question made a visual representation of the triangle's sides appear to be complementary and appear to construct a straight line across their bases while not actually definitively indicating them as such.
The way these triangle's are represented is already skewed so perhaps that is what they are trying to do.
The thing is though, at that point they are defying convention and reasonable assumptions so much that they aren't worth engaging seriously because it's flawed communication.
The version people are choosing to answer seriously is equivalent to a guy holding up a sign that says "ask me about my wiener to get one in a flash for free!" while standing next to a hot dog stand. If you ask he flashes his junk at you and says cheekily "haha you just assumed wrong! Idiot!"
That's already dumb enough but some people could see the clues that suggest he was actually intended to flash people the whole time through a series of reasonable assumptions about his outfit lacking pants or the hit dog stand not even being turned on.
Your argument that we can't assume the line at the bottom is straight is like saying we can't assume the theoretical trenchcoat man won't toss a rabid dachshund he was hiding under the coat at us because the hot dog stand has no buns or condiments on it.
You might not be provably wrong but it's really not worth thinking like an insane person just because a few conventions were defied
So why do you assume the diagram depicts Euclidian space? Why do you assume the numbers are base 10? Why do you assume the question is in English, not just some language that looks like English but has different semantics?